Unit 1 Describing Data
2.4 Measures of Variation
Range, Variance, Standard Deviation
In this course you will encounter 2 different uses of the Greek letter sigma.
Upper case sigma \( \begin{equation} \sum=\end{equation}\) sum
Lower case sigma \(\begin{equation} \sigma= \end{equation}\) standard deviation
Range:
Difference between the minimum and maximum values in the data x max - x minVERY sensitive to extreme values. Can increase dramatically with the inclusion of one or more outliers.
Standard Deviation:
A measure of variation of all values from the mean.- The units are the same as the units of the data.
- If all your data are the same value, your standard deviation will be zero.
- It the sample is small and the range is large, \(s\) can increase dramatically with the inclusion of one or more outliers.
sample standard deviation is a statistic
\( \begin{equation} s=\sqrt{\frac{\sum(x-\bar{x})^{2}}{(n-1)}} \end{equation}\)\(\begin{equation} s=\sqrt{\frac{\left(n \sum x^{2}-\left(\sum x\right)^{2}\right)}{n(n-1)}} \end{equation}\)
population standard deviation is a parameter
\(\begin{equation} \sigma=\sqrt{\frac{\sum(x-\mu)^{2}}{N}} \end{equation}\)
Variance:
A measure of the variation equal to the square of the standard deviation.The sample variance, \(s^2\), is an unbiased estimator of the population variance, \(\sigma^2\).
Range Rule Of Thumb:
minimum usual value = \(\begin{equation} \mu-2 \sigma \end{equation}\)
maximum usual value = \(\begin{equation} \mu+2 \sigma \end{equation}\)
Note: if a sample is NOT REPRESENTATIVE of the population, you can’t tell much about the population center or variation based on the sample.
Calculating Standard Deviation
- Calculate the standard deviation and variance of the following data set using the formulas. Check your answer with technology.
Data Set: 3 5 6 7 9
\(\begin{equation} \bar{x}_{A}=6 \quad n_{A}=5 \quad s_{A}=2.24 \end{equation}\)
\(s_{A}=\sqrt{\frac{\sum(x-\overline{x})^{2}}{n-1}}=\sqrt{\frac{(3-6)^{2}+(5-6)^{2}+(6-6)^{2}+(7-6)^{2}+(9-6)^{2}}{5-1}}=\sqrt{\frac{9+1+0+1+9}{4}}=\sqrt{\frac{20}{4}}=\sqrt{5} \approx 2.24\)
- Suppose that Samira records the ages of customers at the Snack Bar on a college campus from 12-2pm tomorrow, while Mary records the ages of customers at the McDonald’s near the highway at the same time. Who will have the larger SD of their ages – Samira or Mary? Explain why.
Mary will have the larger standard deviation because there is more likely to be a greater variation of ages for the customers at the McDonalds on the highway compared to the ages of the customers at the college snack bar.
- Compare and contrast the following data sets. Given the standard deviation of Graph 1 is approximately 4.8, estimate the standard deviations of graphs 2-4. Record your observations.

Graph 1: Mean=5, Median=5
Standard Deviation=4.77
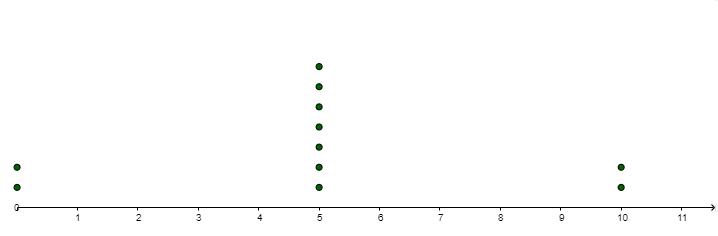
Graph 2: Mean=5, Median=5
Standard Deviation=3.02
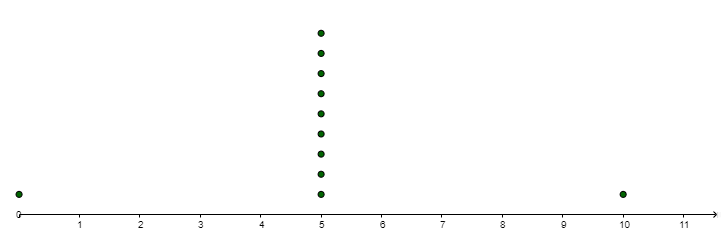
Graph 3: Mean=5, Median=5
Standard Deviation=2.13
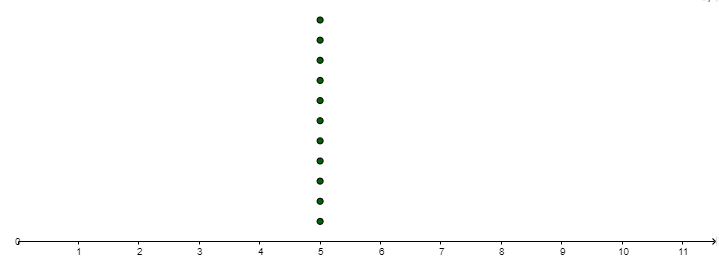
Graph 4: Mean=5, Median=5
Standard Deviation=0
- The graphs below show the results for the medal race of the mens' 400 meters at the 2012 and 2016 games. What do you notice about these times? How are the times from the two years similar? How are they different? Which year do you think had a more "exciting" race? Explain.
- Fuel Economy: A sample of midsized cars have the given fuel efficiencies (Combined City/Highway MPG). Use the sample data below to answer the questions.
(Data from fueleconomy.gov)
23 20 58 32 12 26 27 20 40 24- Find the max and min USUAL values. Sketch the number line and shade the unusual regions.
Using Technology Mean, \(\bar{x}=28.2\) Standard Deviation, \(s=12.9\)
Minimum USUAL value = \(\bar{x}-2s=28.2-2(12.9)=2.4\)
Maximum USUAL value = \(\bar{x}+2s=28.2+2(12.9)=54\)

- Is the value of 58 MPG unusual?
Yes - Is the value of 28 MPG unusual?
No
- Find the max and min USUAL values. Sketch the number line and shade the unusual regions.
- Load the data set from StatCrunch Agricultural Land Percentages. The amounts of agricultural land (% of land area) for a sample of countries are listed.
(2009 Data from World Bank)
Link to accessible version of the Agricultural Land Percentages Accessible Agricultural Land Percentages
- Find the max and min USUAL values. Sketch the number line and shade the unusual regions:
Using Technology Mean, \(\overline{x}=38.14\) Standard Deviation, \(s=22.81\)
Minimum usual value = \(38.14 - 2(22.81) = -7.48\)
Maximum usual value = \(38.14 + 2(22.81) = 83.76\)
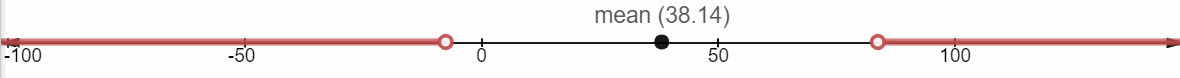
- Are there any unusual values in the data?
Yes, 84.6 Uruguay
- Find the max and min USUAL values. Sketch the number line and shade the unusual regions:
- Estimate the mean and standard deviation of the following frequency distribution using the formulas above. Confirm your answers using technology.
Class Frequency 0 - 8 6 9 - 17 10 18 - 26 5 27 - 35 3 36 - 44 1
\(\bar{x}=\frac{(4 \times 6)+(13 \times 10)+(22 \times 5)+(31 \times 3)+(40 \times 1)}{25} \approx 15.88\)
\(s=\sqrt{\frac{\left((4-15.88)^{2} \times 6\right)+\left((13-15.88)^{2} \times 10\right)+\left((22-15.88)^{2} \times 5\right)+\left((31-15.88)^{2} \times 3\right)+\left((40-15.88)^{2} \times 1\right)}{25-1}}\)
\(s \approx \sqrt{\frac{2384.64}{24}} \approx \sqrt{99.36} \approx 9.9679\)
- Estimate the mean and standard deviation for the age of a U.S. President at the time of inauguration. (This is ALL the U.S. presidents, so this is a population. You need to use the unadjusted variance and standard deviation.)
Age Frequency 40 - 44 2 45 - 49 7 50 - 54 13 55 - 59 12 60 - 64 7 65 - 69 3 70 - 74 1 \(\mu = 55.1\) years
\(\sigma = 6.7\) years.
Note, the sample standard deviation would be \(s = 6.8\) years. You must calculate the unadjusted standard deviation for a population dataset.
- Using technology, estimate the mean and standard deviation for one student’s grade information from their first year of college.
Grade Credit Hours A 9 B 6 C 14 D 0 F 3 \(\overline{x} = 2.6\)
\(s = 1.2\)

-
IQ SCORES: IQ scores have a bell-shaped distribution with a mean of 100 (\(\mu = 100\)) and a standard deviation of 15 (\(\sigma = 15\)). What percentage of IQ scores are:


- Between 70 and 130?
\(13.5+34+34+13.5=95 \%\) - Below 115?
\(0.15+2.35+13.5+34+34=84 \%\) - Above 70?
\(13.5+34+34+13.5+2.35+0.15=97.5 \%\) - Between 85 and 130?
\(34+34+13.5=81.5 \%\) - Usual Values are between and .
\(70 \text { and } 130\) Mimimum usual value: \(\mu-2 \sigma=100-2(15)=70\)
Maximum usual value: \(\mu+2 \sigma=100+2(15)=130\)
- Between 70 and 130?
- Sketchy Milk Company: A dairy fills gallons of milk with a mean of 125.0 fluid ounces and a standard deviation of 0.3 fluid ounces. The volumes have a bell-shaped distribution. Using the empirical rule, what is the approximate percentage of milk amounts:

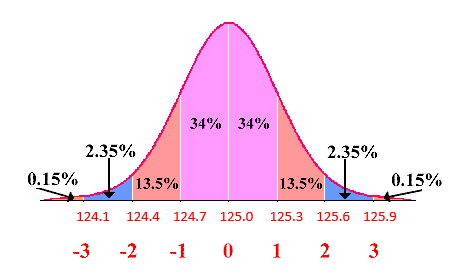
- Between 124.4 fluid ounces and 125.6 fluid ounces?
\(13.5+34+34+13.5=95 \%\) - Below 124.7 fluid ounces?
\(0.15+2.35+13.5=16 \%\) - Above 124.7 fluid ounces?
\(34+34+13.5+2.35+0.15=84 \%\) - Between 125.0 fluid ounces and 125.6 fluid ounces?
\(34+13.5=47.5 \%\) - Usual values are between and ?
\(124.4 \text { and } 125.6\) Minimum usual value: \(\mu-2 \sigma=125.0-2(0.3)=124.4\) fluid ounces
Maximum usual value: \(\mu+2 \sigma=125.0+2(0.3)=125.6\) fluid ounces
- Between 124.4 fluid ounces and 125.6 fluid ounces?
What was the mean time in each of the 2 years? On the dotplots shown, sketch vertical lines which you feel best represent the mean for each year.
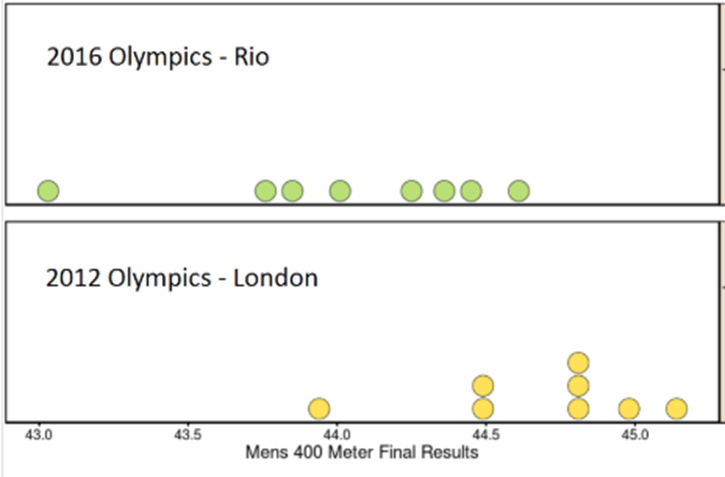
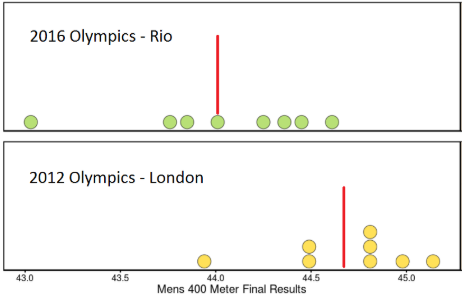
The standard deviations from each year are: 2016: 0.471 seconds 2012: 0.349 seconds. Explain to somebody who has never seen standard deviations before how these numbers allow us to compare the results from 2012 and 2016.
2016's higher standard deviation shows the 8 runners' times are individually farther from the mean (from "average").
Using Standard Deviation to Identify Unusual Values in the Data Set
Calculating Measures of Variations in Binned Data
Mean: \(\bar{x}=\frac{\sum x_{i} f_{i}}{\sum f}\)
Standard Deviation \(s=\sqrt{\frac{\sum\left(x_{i}-\overline{x}\right)^{2} f_{i}}{n-1}}\)
The Empirical (68-95-99.7) Rule for Data With a Bell Shaped Distribution
ABOUT 68% of all the values fall within 1 standard deviation of the mean. \(\mu \pm \sigma \)
ABOUT 95% of all the values fall within 2 standard deviations of the mean. (the other 5% are unusual) \(\mu \pm 2 \sigma\)
ABOUT 99.7% of all the values fall within 3 standard deviations of the mean. \(\mu \pm 3 \sigma\)
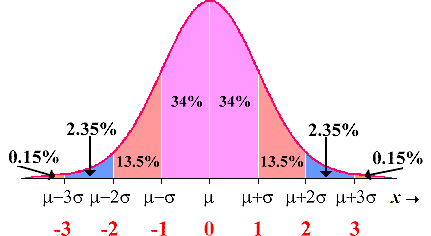
As an example, assume \(\mu=10 \text { and } \sigma=2\)
Maximum usual value \(=\mu+2 \sigma=10+2(2)=14\)
Minimum usual value \(=\mu-2 \sigma=10-2(2)=6\)