Math 1010

Linear Modeling and Logic Unit

4.9 Using Venn Diagrams to Analyze Deductive Arguments

State the premise illustrated by each Venn diagram

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents the things that Tasha likes and the small circle represents dogs.

Tasha loves all dogs.

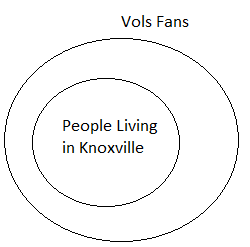
A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents Vols fans and the small circle represents people living in Knoxville.

All people living in Knoxville are Vols fans.

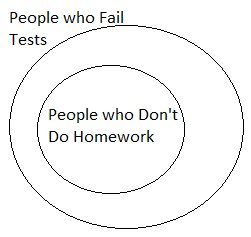
A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents people who fail tests and the small circle represents people who don't do homework.

All students who don't do their homework fail tests.

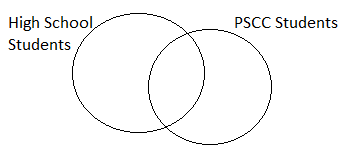
A Venn Diagram with two intersecting circles. One circle represents high scool students and the other circle represents PSCC students.

Some high school students are PSCC students.

A Venn Diagram with two disjoint circles. One circle represents Rolls Royce cars and the other circle represents cars in the PSCC parking lot.

No cars parked in the PSCC parking lot are Rolls Royce cars.

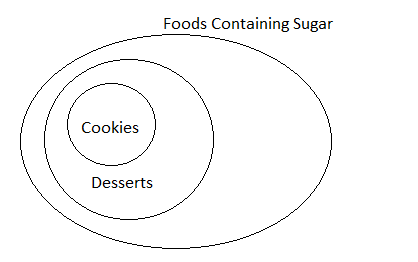
A Venn Diagram with a large circle. A small circle is completely inside the large circle and an even smaller circle is completely inside the small circle. The large circle represents foods containing sugar. The next size circle represents desserts and the smallest circle represents cookies.

All cookies are desserts and foods containing sugar.

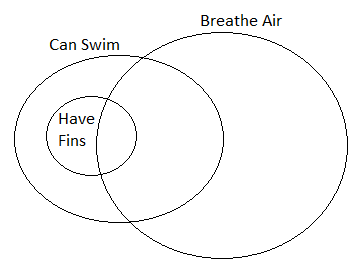
A Venn Diagram with three intersecting circles. A small circle representing creatures that have fins is completely inside a circle that repsents creatures that can swim. The largest circle, creatures that breathe air, intersects both of the other two circles.

All creatures that have fins can swim.

Some creatures that breathe air can swim.

Some creatures that breathe air can swim and have fins.

Deductive Argument: makes a case for a specific conclusion from a more general premise

Valid if the conclusion follows necessarily from its premises (even if premises aren’t true)
Sound if the argument is valid AND the premises are true.

Decide if the arguments are valid based on the premises illustrated by the Venn diagrams in #1-7.

Use Venn diagram #1
1. Lucky is a dog, therefore Tasha loves Lucky.
2. Lucky is a four leaf clover, therefore Tasha does not love Lucky.
Use Venn diagram #2
1. Lara lives in Knoxville, therefore Lara is a Vols fan.
2. Lara is a Vols fan, therefore Lara lives in Knoxville.
Use Venn diagram #3
1. You didn’t do your homework, therefore you failed the math test.
2. You did your homework, therefore you passed the math test.
Use Venn diagram #4
1. Daniel is in high school, therefore Daniel does not take classes at PSCC.
2. Daniel is not in high school, therefore Daniel is a student at PSCC.
Use Venn diagram #5
1. I drive a Rolls Royce, therefore I don’t park in the PSCC parking lot.
2. I drive a Honda, therefore my car is in the PSCC parking lot.
Use Venn diagram #6: Snickerdoodles are cookies, therefore they contain sugar.

valid

Use Venn diagram #7
1. Fish have fins, therefore they breathe air.
2. Fish have fins, therefore they can swim.
3. Dogs can swim, therefore they have fins.

The following deductive arguments are called syllogisms. A syllogism is an argument that contains two premises and a conclusion.

Using a Venn diagram, determine if the following arguments are valid or invalid.

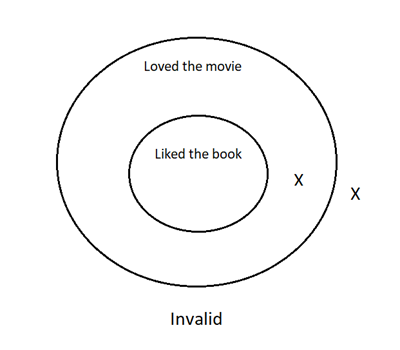
Premise: If you liked the book, then you’ll love the movie.
Premise: You did not like the book.

Conclusion: You will not love the movie.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents those who loved the movies and the small circle represents those who liked the book. There is an X inside the large circle, but not inside the small circle. There is also an X outside both circles. The argument is invalid.

Premise: Monocot flower parts are in multiples of three.
Premise: Apple flowers have five petals.

Conclusion: Apple trees are not monocots.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents flowers that are multiples of three. The small circle represents monocots. There is an X outside both of the circles. The argument is valid.

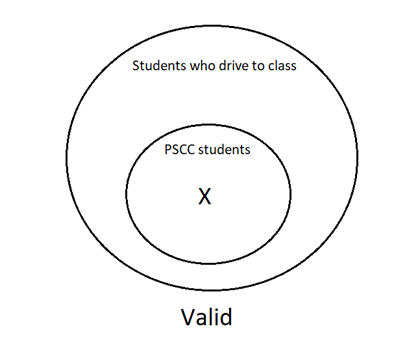
Premise: All Pellissippi State students drive to class.
Premise: Sarah is a Pellissippi State student.

Conclusion: Sarah drives to class.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents students who drive to class. The small circle represents all PSCC students. There is an X inside the PSCC students' circle. The argument is valid.

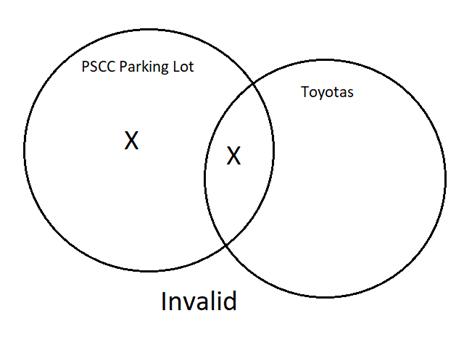
Premise: Some of the cars in the PSCC parking lot are Toyotas.
Premise: Emily’s car is in the PSCC parking lot.

Conclusion: Emily’s car is a Toyota.

A Venn Diagram with two intersecting circles. One circle represents the PSCC parking lot. The other circle represents Toyotas. There is an X in the intersection of the circles and another X in the PSCC parking lot circle that is not part of the intersection. The argument is invalid.

Premise: All dairy products contain protein.
Premise: Soybeans contain protein.

Conclusion: Soybeans are dairy products.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents things that contain protein. The small circle represents dairy products. There is one X inside the dairy products circle and one X in the large circle but not in the dairy products circle. The argument is invalid.

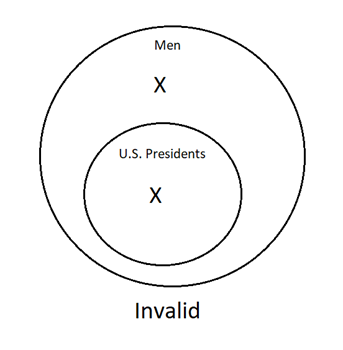
Premise: All U.S. Presidents have been men.
Premise: George Washington was a man.

Conclusion: George Washington was a U.S. President.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents men and the small circle represents U.S. Presidents. There is one X inside the U.S. Presidents circle and one X inside the Men circle but not in the U.S. Presidents circle. The argument is invalid.

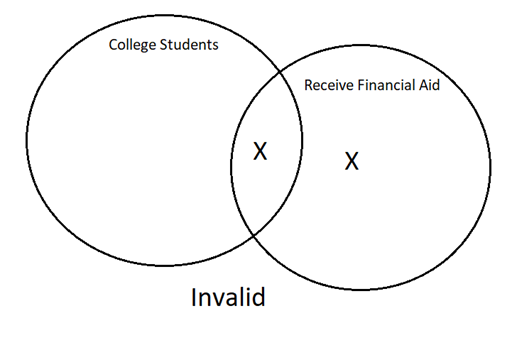
Premise: Some college students receive financial aid.
Premise: Cara is receiving financial aid.

Conclusion: Cara is a college student.

A Venn Diagram of two intersecting circles. One circle represents colege students and the other represents those who receive financial aid. There is an X inside the intersection of the circles and there is another X in the receive financial aid circle but not in the college students circle. The argument is invalid.

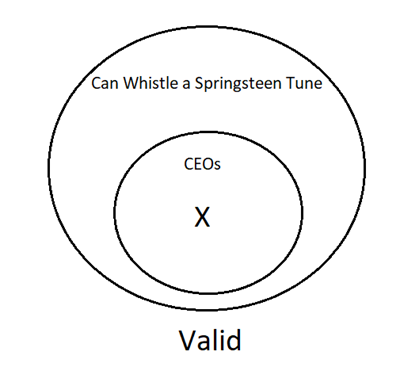
Premise: All CEOs can whistle a Springsteen tune.
Premise: Steve Jobs was a CEO.

Conclusion: Steve Jobs could whistle a Springsteen tune.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents those who can whistle a Springsteen tune and the small circle represents CEOs. The is an X inside the CEO circle. The argument is valid.

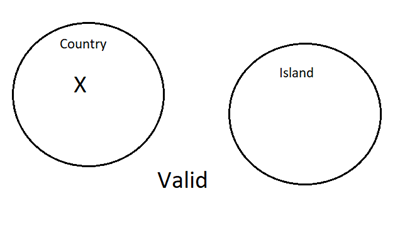
Premise: No country is an island.
Premise: Iceland is a country.

Conclusion: Iceland is not an island.

A Venn Diagram with two disjoint circles. One circle represents Countrys and the other circle represents Islands. There is an X inside the country circle and there is an X outside of both circles. The argument is valid.

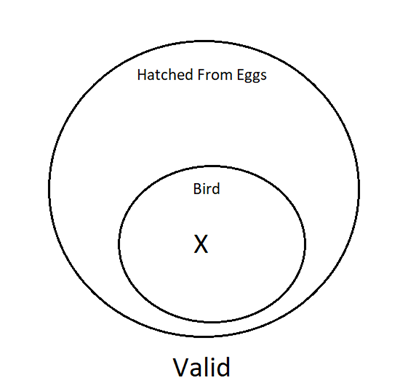
Premise: If it is a bird, then its young are hatched from eggs.
Premise: Condors are birds.

Conclusion: Condor chicks are hatched from eggs.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents young that are hatched from eggs. The small circle represents birds. There is an X in the bird circle. The argument is valid.

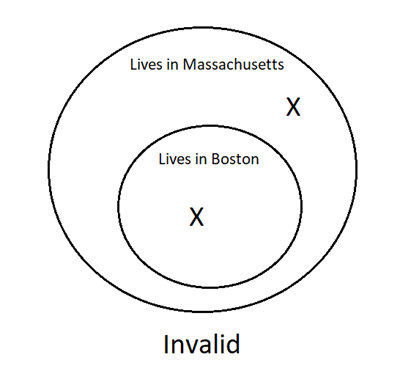
Premise: If you live in Boston, you live in Massachusetts.
Premise: Bruno lives in Massachusetts.

Conclusion: Bruno lives in Boston.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents people who live in Massachusetts. The small circle represents people who live in Boston. There is one X inside the live in Boston circle and one X in the live in Massachusetts circle that is not in the live in Boston circle. The argument is invalid.

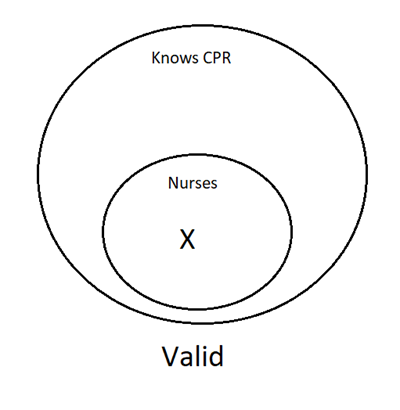
Premise: It’s necessary for nurses to know CPR.
Premise: Tom is a nurse.

Conclusion: Tom knows CPR.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents people who know CPR and the small circle represents nurses. There is an X inside the nurses circle. The argument is valid.

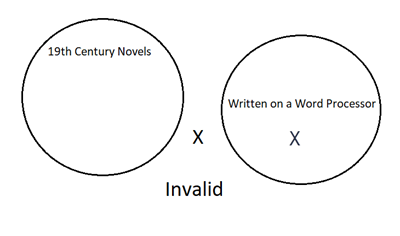
Premise: Novels written in the 19th century were not written on a word processor.
Premise: Jake finished writing his first novel last year.

Conclusion: Jake’s first novel was written on a word processor.

A Venn Diagram with two disjoint circles. One circle represents 19th century novels and the other circle represents novels written on a word processor. There is an X outside both circles. The argument is invalid.

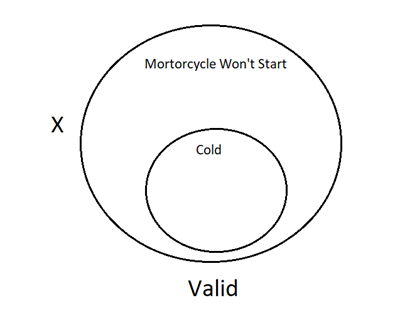
Premise: If it is cold, my motorcycle will not start.
Premise: My motorcycle started.

Conclusion: It is not cold.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents that the motorcycle won't start. The small circle represents that it is cold. There is an X outside both circles. The argument is valid.

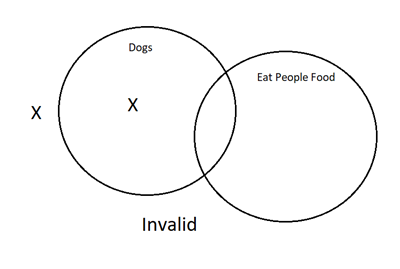
Premise: Some dogs eat people food.
Premise: Callie eats dog food.

Conclusion: Callie is not a dog.

A Venn Diagram with two intersecting circles. One circle represents dogs and the other circle represents those who eat people food. There is an X inside the dogs circle but not in the intersection. There is another X outside both circles. The argument is invalid.

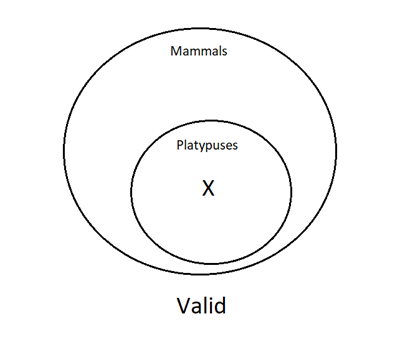
Premise: Platypuses are mammals.
Premise: Platypuses lay eggs.

Conclusion: Some mammals lay eggs.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents mammals and the small circle represents platypuses. There is an X inside the platypus circle. The argument is valid.

Premise: If it rains or snows, then I read.
Premise: I am not reading.

Conclusion: It is neither raining or snowing.

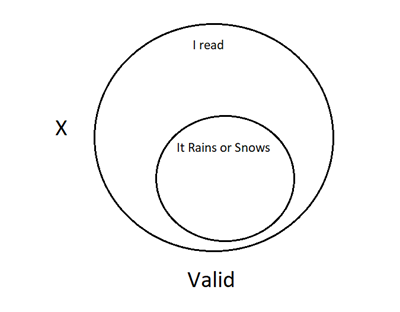
A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents that I read. The small circle represents that it rains or snows. There is an X outside both circles. The argument is valid.

Premise: If it rains or snows, then I read.
Premise: I am reading.

Conclusion: It is raining or snowing.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents that I read. The small circle represents that it rains or snows. There is one X inside the small circle and another X inside the large circle, but not inside the small one. The argument is invalid.

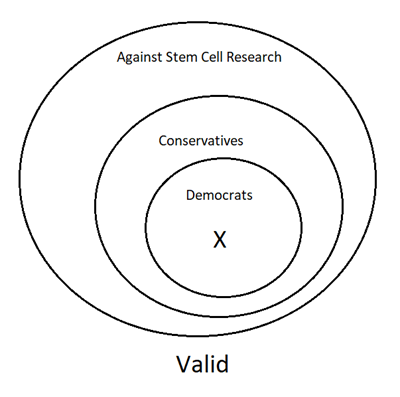
Premise: All Democrats are conservatives.
Premise: All conservatives are against stem cell research.

Conclusion: All Democrats are against stem cell research.

A Venn Diagram of three nesting circles. The largest circle represents anyone who is against stem cell research. The next size circle represents conservatives and the small circle represents Democrats. There is an X inside the Democrats circle. The argument is valid.

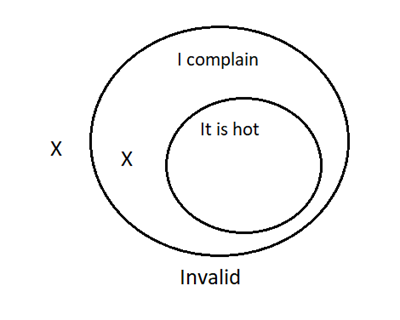
Premise: I always complain when it’s hot.
Premise: It’s not hot.

Conclusion: I’m not complaining.

A Venn Diagram with one smaller circle completely inside the larger circle. The large circle represents that it is hot. The small circle represents that I complain. There is an X outside both circles. The argument is valid.