Statistics Unit

2.3 Interpreting Graphs

Introduction

In mathematics classes, you will learn how to draw many different types of graphs, from linear and quadratic graphs to graphs of trigonometric functions if you take Precalculus. When drawing these graphs, it is sometimes fairly easy to get the points plotted and connect them appropriately, but it is more difficult to explain what the points and other parts of the graph mean. Graphs are not just a bunch of random points placed on a grid to form a line or curve. Each point on the graph has a specific meaning in context of the stated problem. In this lesson, you will gain a firmer understanding of how to look at parts of a graph and give a clear interpretation of what each part means within the context of the problem.

The graph below is called a scatter plot of points. A scatter plot is a collection of points showing the relationship between two variables. Sometimes, a trend may be recognized by these points, but at other times, there may be no trend as in the example below.

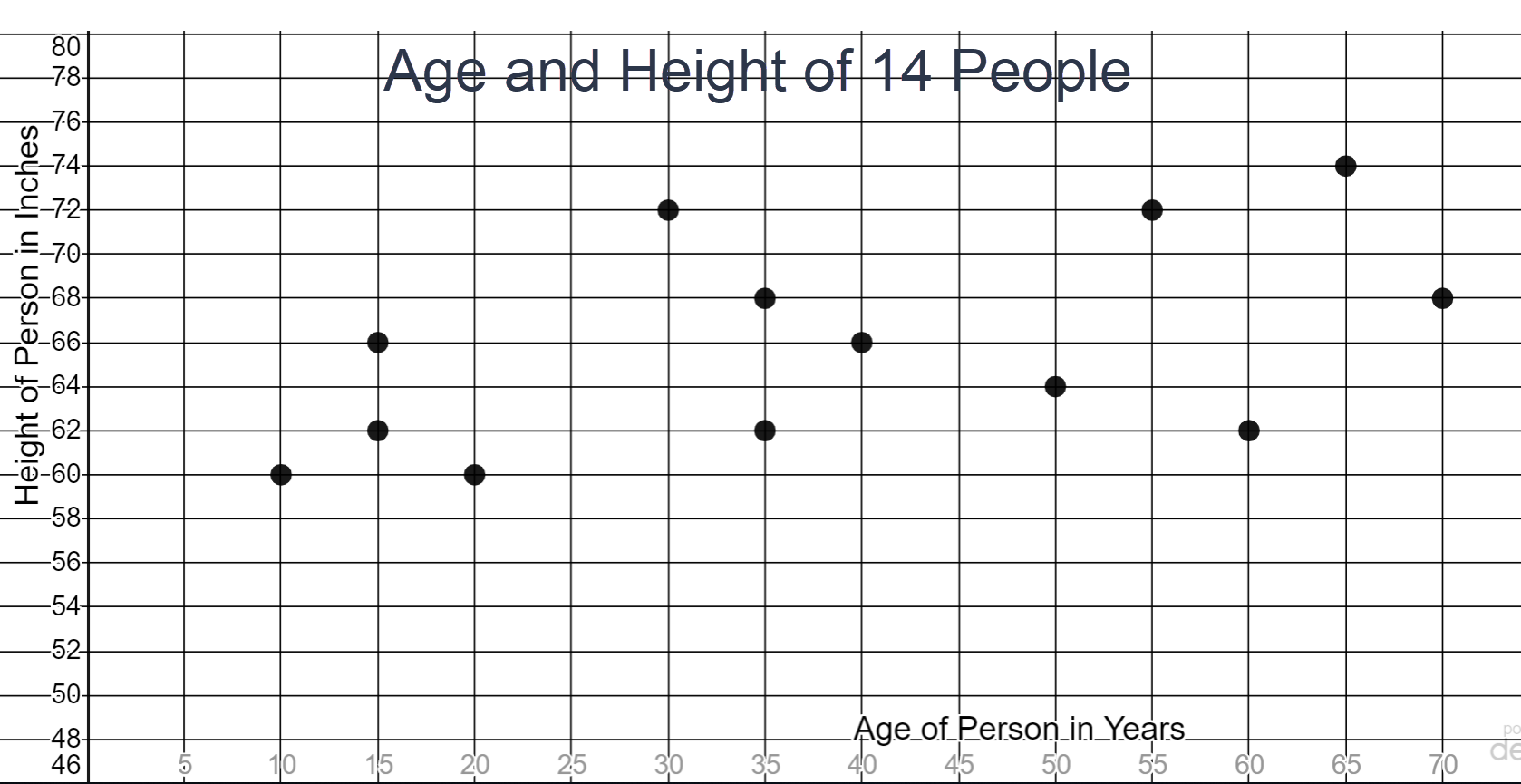
The graph is a scatter plot of points. The x-axis represents the age of each person in years and the y-axis represents the height of each person in inches. the points that are plotted on the graph are (10,60), (15,62), (15,66), (20,60), (20,76), (30,72), (35,62), (35,68), (40,66), (50,64), (55,72), (60,62), (65,74), (70,68).

  1. In this problem, 14 people were asked their age and their height was measured. Answer the following questions based on the graph.

    1. Only one person surveyed was 40 years old. How tall is this person? ________________

      2. 66 inches

    2. Three people were 62 inches tall. How old were the three people? ________________________

      3. 15, 35 and 60 years old

    3. One point represented in the graph has the coordinates (55,72). Explain the meaning of this point within the context of this graph. Use a complete sentence for your explanation.

      4. A 55 year old in the survey is 72 inches tall.

  2. Katie is planning to play Putt Putt with her friends. The Putt Putt course in her town charges $8.00 to rent a club and ball but then only $3.00 for every 18-hole game she golfs. The equation that models this situation is \(y=8.00+3.00x\) where x represents the number of games played and y represents the total cost for Katie to golf. A table of values and a graph for this problem are pictured below.

    Games Cost (in dollars)
    x 8.00+3.00x
    1 11
    2 14
    5 23
    8 32
    10 38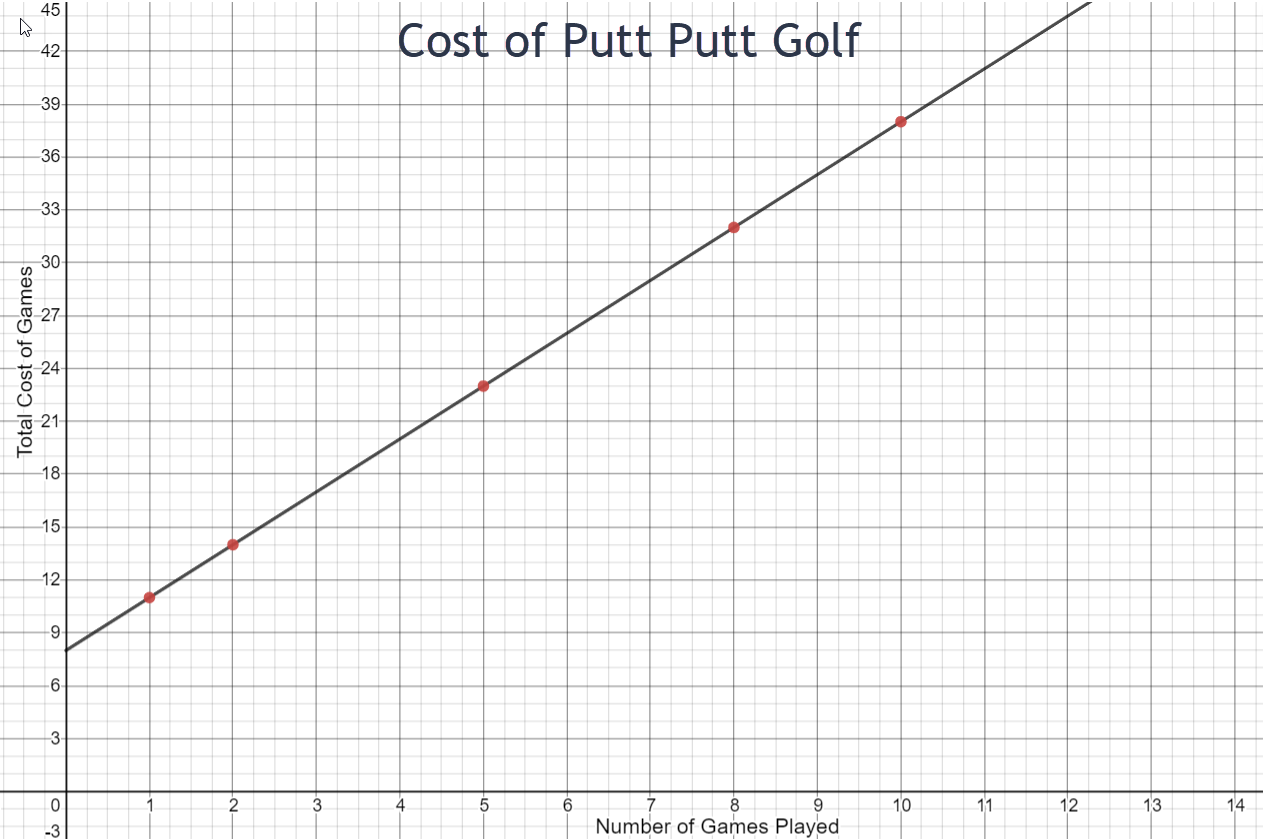

    The graph is linear. The x-axis represents the number of games golfed and goes from 0 to 15. The y-axis represents the total cost of the games and goes from 0 to 45. There are five points plotted on the line. They are (1,11), (2,14), (5,23), (8,32) and (10,38).

      Answer the following questions based on the graph.

    1. What would Katie’s total cost be if she played 12 games? _________________

      2. \(8 + 3 \cdot 12 = \$44\)

    2. If Katie paid $26.00 to golf, how many games did she play? _______________

      3. 6 games

    3. The point (3,17) lies on this graph. Explain what this point means within the context of this problem.

      4. Katie pays $17 to play 3 games.

    4. The graph of this line is rising from left to right. In a complete sentence, explain why this is happening.

      5. The cost increases as the number of games played increases.

Group Assignment

Study each of the pictured graphs and answer the questions that follow each graph. Be sure to use a complete sentence in your answers where appropriate.

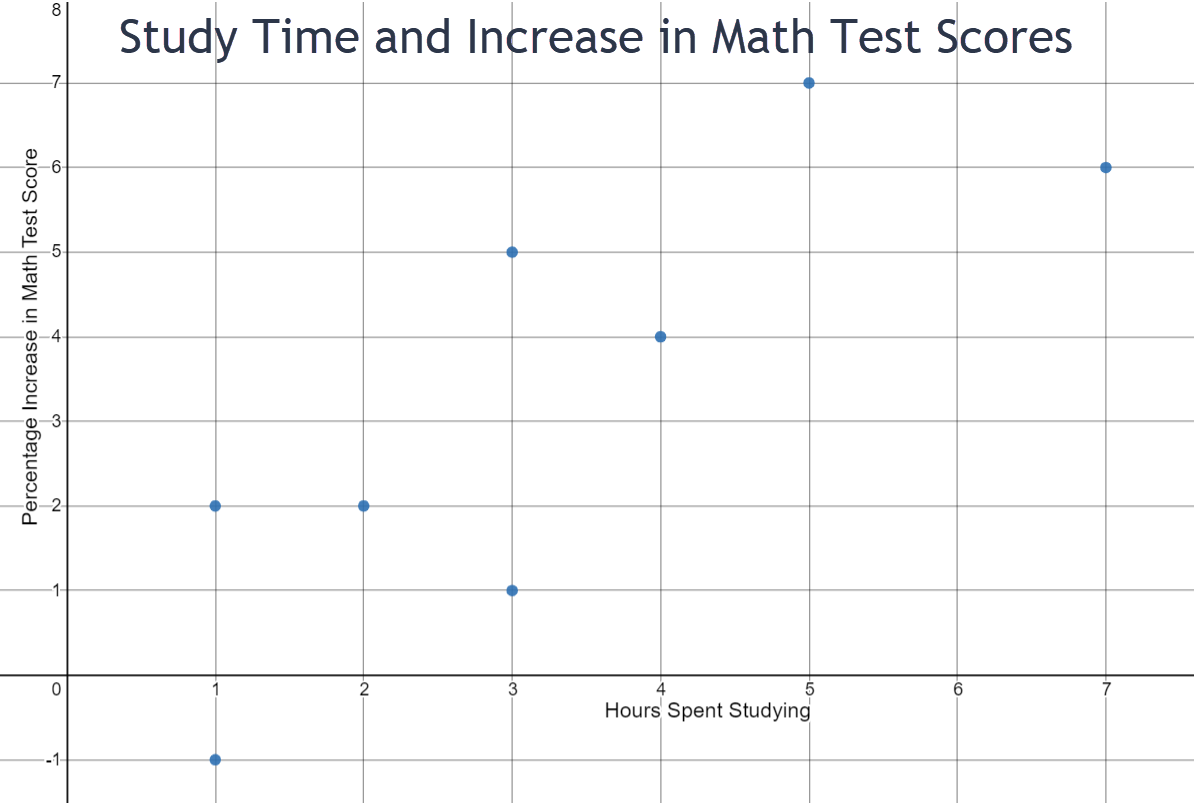
The graph is a scatter plot of points. The x-axis represents the hours spent studying and goes from 0 to 7. The y-axis represents the percent increase in the math test score and goes from -6 to 8. The points plotted on the graph are (1,-1), (1,2), (2,2), (3,1), (3,5), (4,4), (5,7), (6,1) and (7,6).

  1. Use the scatter plot above to answer the following questions.
    1. What is being measured on the horizontal axis?

      2. Number of hours spent studying

    2. What is being measured on the vertical axis?

      3. Percentage increase in math test score

    3. The point (5,7) lies on the graph. Explain what this point means within the context of the graph.

      4. A student who spent 5 hours studying had a 7% increase on her math test score.

    4. Two students in the sample studied for 3 hours. What were their percent increases in their math test scores? State your answer as a complete sentence.

      5. One student who studied three hours increased her test score by nearly 1%. The other student who studied for 3 hours increased her test score by approximately 5%.

    5. How many hours did one student study whose test score increased by 2 percent? Use a complete sentence in your explanation.

      6. One student whose test score increased by 2% studied for 1 hour.

    6. Do you see a relationship between the amount of time that a student studies and the percentage of increase in the math test score? Explain the relationship in a complete sentence.

      7. The trend seems to be that the more you study, the higher increase in your math test score.

    7. Is (-1,-5) a valid point that could be on this graph? Explain your answer in a complete sentence.

      8. No. A student cannot study for a negative number of hours.

  2. In 2021, the average cost for thirty seconds of prime-time television advertising time was $120,000. The equation that models this situation is

    \(y=120000x\)

    where x is the number of 30-second ads and y is the cost of each advertisement. The graph is a linear graph and is shown below.

    The graph is linear and increases from left to right. The x-axis represents the number of minutes in advertising time and goes from 0 to 11. The y-axis represents the cost of the advertisements and goes from 0 to 1,500,000. The graph is constructed according to the equation y=120000x.
    1. If a company buys 6 30-second ads, what is the approximate cost of the advertisements? State your answer in a complete sentence.

      2. The approximate cost of a 6 30-second ads is $720,000

    2. If the cost of one ad buy is approximately $500,000, how many 30-second ads were bought? State your answer in a complete sentence.

      3. A $500,000 ad is approximately 4 30-second ads.

    3. What is the cost of a twelve-minute advertisement? How did you find this answer?

      4. \(y = 120,000 \cdot 12 = $1,440,000\)

    4. The graph is increasing from left to right. In a complete sentence, explain this.

      5. As the number of 30-second ads increases, the cost of the ad increases.

    5. The point (-2, -240000) would lie on this line if the bounds for the x-axis and the y-axis included negative numbers. Is this a valid point for this situation? In a complete sentence, explain why or why not.

      6. No. An ad cannot run for -1 minutes and an ad cannot cost a negative amount of money.

  3. Green Bulk is an internet wholesaler where dried fruits and nuts can be bought in large quantities for use by grocery stores, gourmet markets, coffee shops, and other small businesses. The manager of one specialty store buys 20 cases of organic dried cranberries. Each case contains 25 pounds of dried cranberries. The equation that models this situation is \(y=500-25x\) where x represents the number of cases of dried cranberries that are sold at the specialty store and y represents the number of pounds of dried cranberries that are still in stock at the specialty store. The graph is a linear graph and is shown below. 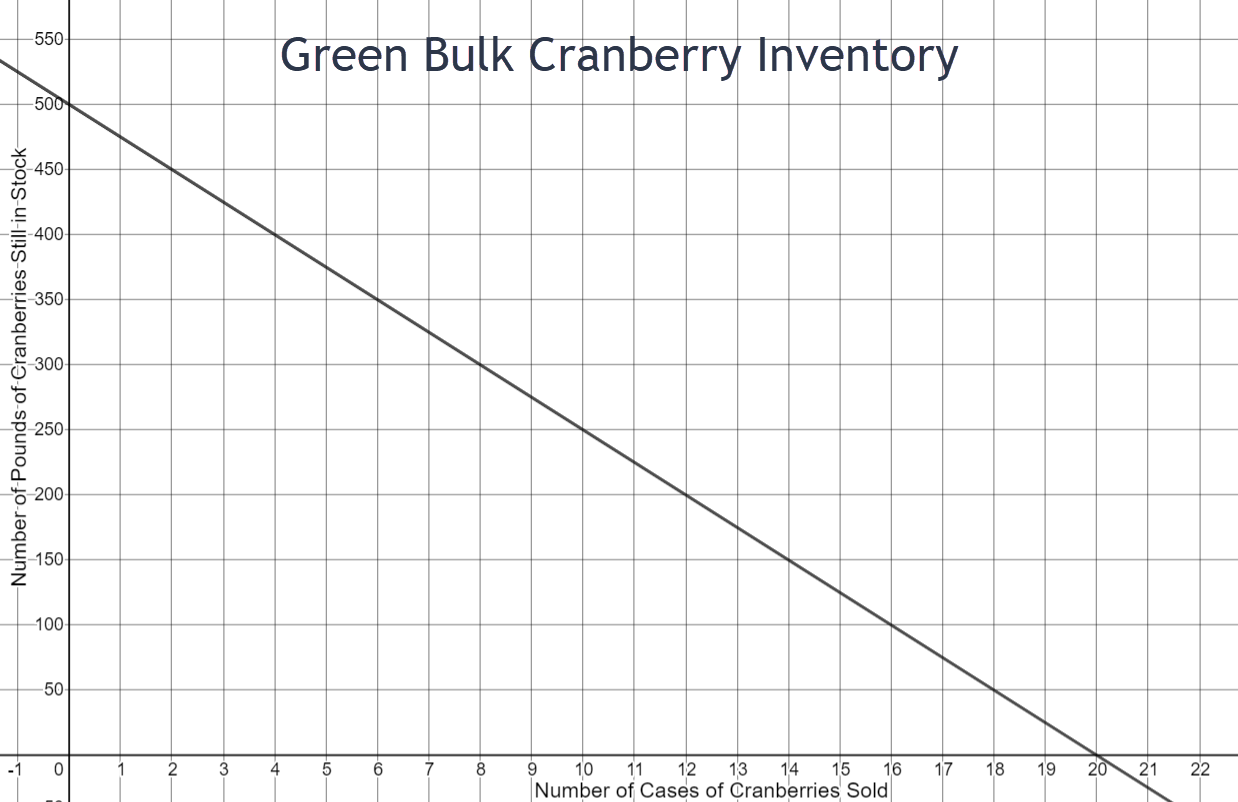The graph is linear and decreases from left to right. The x-axis represents the number of cases of cranberries that are sold and goes from 0 to 24. the y-axis represents the number of pounds of cranberries still in stock and goes from 0 to 600. The line is constructed according to the equation 500-25x. Two points on the graph are (4,400) and (16,100)
    1. If the store sells 16 cases of cranberries, how many pounds of cranberries are still in stock? Use a complete sentence in your answer.

      2. There are 100 pounds of cranberries in stock when 16 cases are sold.

    2. If the store sells 8 cases of cranberries, how many cases of cranberries are still in stock? Use a complete sentence in your answer.

      3. There are 12 cases of cranberries still in stock when 8 cases are sold.

    3. If the store has 500 pounds of cranberries, how many cases does he have in stock and how many cases has he sold? Use a complete sentence in your answer.

      4. If the store has 500 pounds of cranberries, none of the stock was sold so all 20 cases are still left.

    4. The graph is falling from left to right. Use a complete sentence to explain the reason for this.

      5. As the number of cases sold increases, the amount of cranberries in stock decreases.

    5. The point (24,-100) fits the equation that models this situation. Is it an appropriate point for this graph? Use a complete sentence to explain why or why not.

      6. No. Once 20 cases are sold, the stock is gone.

    6. Are there any valid points that would lie on this graph that are beyond the boundaries of the graph as it is currently drawn? If the answer is yes, please give an example and explain what it means within the context of this problem. If the answer is no, please use a complete sentence to explain your answer.

      7. No. There are 20 cases available at the beginning and nothing left when 20 cases are sold. The cranberries could be on back order.

  4. A recent graduate of dental school has investigated two different options for her future. She could go to work for a large organization, which will provide her with an office and all that is needed to go with it, or she could start her own business. She has been told that if she chooses the first option, after taxes she could expect to make about $30,000 per year. She learns, however, if she decides to start her own business, she will need an initial investment of $275,000 to buy an office and the equipment needed to set up her practice. She could expect, after taxes, staff salaries and benefits, lab costs, interest on her initial investment, and various other annual costs, to make about $45,000 per year. The system of equations that model this situation is

    \(y=30000x\)

    \(y=45000x-275000\)

    where x is the number of years she has worked and y represents her salary for each of the two options. The graph of this system is below.

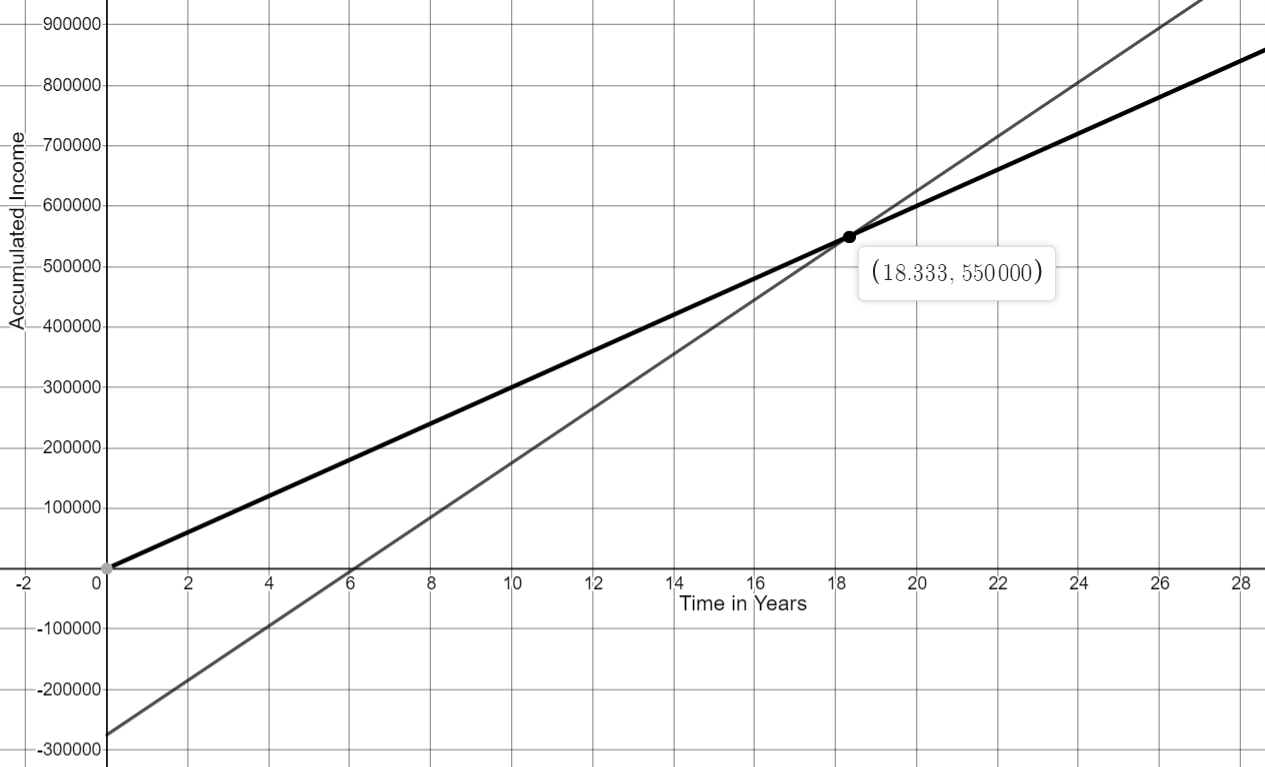
    The graph is two linear equations, each one representing one of the two options the graduate has for employment. The x-axis represents the time in years and goes from 0 to 30. The y-axis represents the accumulated profit and goes from -300,000 to 1,000,000. The two lines are constructed according to the given equations and intersect at the point (18.33, 550,000).
    1. What does the black line (Option A) on this graph represent? What does the gray line (Option B) on this graph represent? Use complete sentences in your answer.

      2. Option A is her first job option in which she would be making about $30,000 per year at a larger organization. Option B is her second job option in which she would start her own business.

    2. The point of intersection of the two lines on this graph is (18.33, 550000). In your own words and using complete sentences, please explain what this point of intersection means within the context of this problem.

      3. If she works for either option for about 18.33 years, she will be making the same amount of money at each, $550,000.

    3. The point (10, 175000) lies on which line? Explain what this point means within the context of this problem. Use a complete sentence in your answer.

      4. If she chooses to start her own business, she will have accumulated $175,000 in her tenth year.

    4. The point (1, 30000) lies on which line? Explain what this point means within the context of this problem. Use a complete sentence in your answer.

      5. If she chooses Option A, she will make $30,000 in her first year.

    5. Is the point (36, 1345000) a valid point for the gray line (Option B)? Explain your answer in a complete sentence.

      6. Yes. After 36 years of working, she will have accumulated $1,345,000.